Electromagnetic Induction Process

The electromagnetic induction process is conceptually summarized in figure 288 from Klein and Lajoie (1980). An EM transmitter outputs a time-varying electric current into a transmitter coil. The current in the transmitter coil generates a magnetic field of the same frequency and phase. Lines of force of this magnetic field penetrate the earth and may penetrate a conductive body. When this occurs, an electromotive force or voltage is set up within the conductor, according to Faraday's Law:

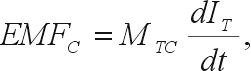(70)

where

Current will flow in the conductor in response to the induced electromotive force. These currents will usually flow through the conductor in planes perpendicular to lines of magnetic field of force from the transmitter, unless restricted by the conductor's geometry. Current flow within the conductor generates a secondary magnetic field whose lines of force, at the conductor, are such that they oppose those of the primary magnetic field. The receiver coil, at some distance from the transmitter coil, is therefore energized by two fields: from the transmitter and from the induced currents in the ground.

Figure 288. Generalized picture of electromagnetic induction prospecting.
(Klein and Lajoie 1980; copyright permission granted by
Northwest Mining Association and Klein)

From Faraday's Law, the EMF induced in the receiver may be expressed as

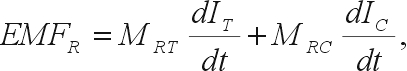(71)

where

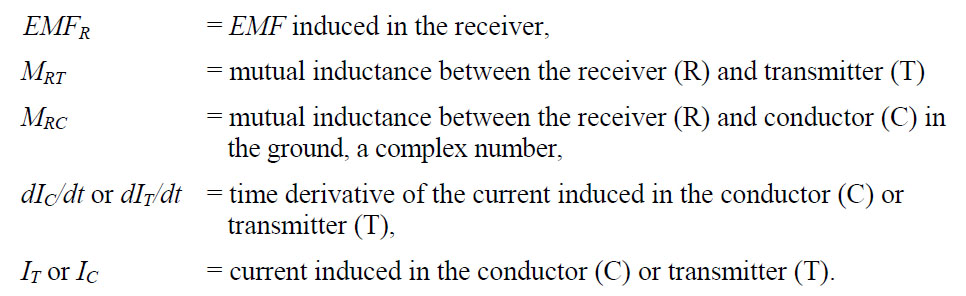
Note that induced currents occur throughout the subsurface, and that the magnitude and distribution are functions of the transmitter frequency, power, and geometry and the distribution of all "electrical properties" in the subsurface, i.e., everything (not just an isolated "conductor"). The above discussion simplifies the problem by assuming the presence of only one conductor embedded in a much less conducting medium.